Warlord Deckbuilding: The Dreaded Hundred Card Emperor
/Emperor Ceihallus is the worst warlord in Ancients. I’m kidding, but The Emperor’s card effect can be paraphrased to “Daybreak: You only draw 3 cards during the draw phase. Nightfall: Do amazing stuff.” His ability to scribe for bonuses and discard for bonuses means that early game resource management is key. In the early game, if you over-commit to charging The Emperor up, you may not be able to stay in the game. If you don’t commit enough, you’re sacrificing advantages that compound over time, thus nullifying his upside.
Facts About Emperor Ceihallus
After building and playing over 15 different Emperor decks, I found a few consistencies, no matter how I built him.
The Emperor loves the late game. The longer the game goes, the better he becomes.
The Emperor is “meh” turn one. Games where The Emperor felt the worst were games where turn one was very impactful for the opponent. For example, games where the opponent could win on turn 1, or enter turn 2 just needing to mop up were worst case scenario for Big ol’ E.
The Emperor doesn’t need a lot of offense to come from behind. One card like Tsunami with a buffed DC can go a long way to equalizing any deficit.
There isn’t any one way to build The Emperor. His ability is a blank canvas. He generates card advantage in the late game by doing things wizards already want to do and he generates explosive turns by having the ability to pop-off two to three spend order spells in one explosive turn.
So I began looking for the best ways to consistently get to the “late game”. I settled on the meatball strategy. Play a deck full of big, meaty, 3 HP ghosts and high AC guys, stun ‘em up, and who cares what comes next. Just stabilize: trust that you can put out more meat than your opponent can gobble up, and that they’ll run out of strikes before you run out of HP. As I began building for this strategy, I conceived my ideal hand: then I hopped onto Stat Trek to check my odds of drawing it.
Expand Your Mind: Expand Your Deck
That's when I had the epiphany. Half your deck at most can be one card type, and 6 characters start in play, so at 50 cards, only about 43% of the cards in your deck are characters. However, when you increase the total number of cards, the amount of characters in play is constant, so those 6 characters have less of a negative effect on the percentage of characters in your deck. Also, including cards like Figurine of Wonder and Living History can inflate that percentage even higher!
I began walking through hypergeometric distribution scenarios. If I only care about my opening hand having as many characters as possible, how should I build my deck? Hypergeometric distribution can be used to calculate the odds of drawing cards based on a few different variables. Population size, sample size, and number of successes in population. For purposes of our exercise, population size becomes deck size -6 ( to represent the cards that start in play). Sample size is always five. Cards like Meet at the Inn, that replace themselves, can be accounted for by lowering the population size, but its slightly messy, and I won’t go into that in this article. Number of successes in a sample is also a variable, we’ll call it X, but our most important outcome for X is ≥ 3. After running a few simulations, I found a few things out very quickly.
The bigger the deck, the better your odds of drawing characters on turn one.
You hit a point of diminishing returns somewhere between 75 and 90 cards in your deck.
When you try to explain to your play test partners why you think you should play test an 80-85 card deck they look at you like you have two heads.
When you explain math to people that are only half listening, their eyes glaze over.
100 cards is funnier and more marketable than 85.
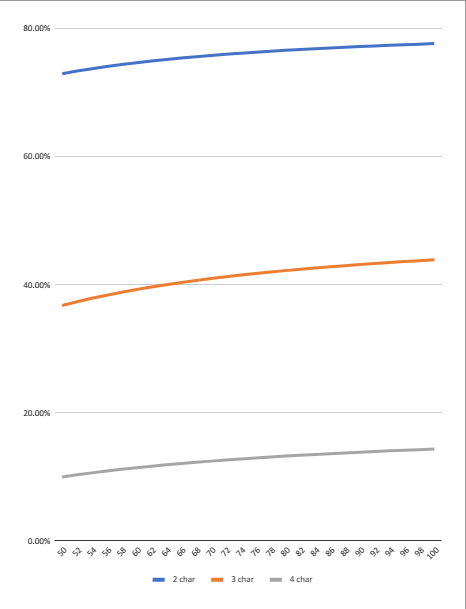
You can see a visual representation of my early work below- with the graph showing the increase in hand probability based on deck size.
Most People Use Only 5% of their Collection to Build a Deck—What if We Used 100?
The odds improvement, especially for our 3 character hand, was very impressive. Over the course of a 5 round tournament, potentially 15 games, I would increase my chance of seeing the hand I wanted by 10%. Could I push the odds a bit?
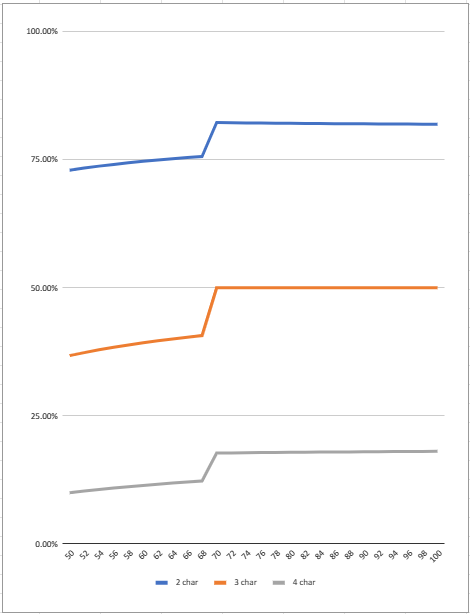
With 100 cards in the deck, I would have room to push things a bit when you get to the 70 card threshold and run cards that simply take up an action or item slot but create a body. Adding in 3 of those would increase the odds even farther: see the graph below.
You can see that as the deck size increases, the probability of each hand size remains the same up until the introduction of the faux characters, which gives us a 50/50 of a 3 or more character hand, and a whopping 80+% chance of drawing at least 2. And our odds of 4 characters goes from the low teens to almost 20%!
Now that we've unraveled the intricate dance between mathematics and deckbuilding, it's time to embrace the wild and wonderful world of numbers! Let's break free from the shackles of conventional thinking and dare to be bold in our card choices. So, fellow players, I implore you to grab your calculators and dive headfirst into the realm of probability and statistics. Let's challenge the status quo, experiment fearlessly, and forge our own paths to triumph. Are you ready to embark on this mathemagical journey? The Emperor awaits your answer.